No CrossRef data available.
Article contents
Effective results for unit points on curves over finitely generated domains
Published online by Cambridge University Press: 13 January 2015
Abstract
Let A be a commutative domain of characteristic 0 which is finitely generated over ℤ as a ℤ-algebra. Denote by A* the unit group of A and by K the algebraic closure of the quotient field K of A. We shall prove effective finiteness results for the elements of the set
where F(X, Y) is a non-constant polynomial with coefficients in A which is not divisible over K by any polynomial of the form XmYn - α or Xm - α Yn, with m, n ∈ ℤ⩾0, max(m, n) > 0, α ∈ K*. This result is a common generalisation of effective results of Evertse and Győry [12] on S-unit equations over finitely generated domains, of Bombieri and Gubler [5] on the equation F(x, y) = 0 over S-units of number fields, and it is an effective version of Lang's general but ineffective theorem [20] on this equation over finitely generated domains. The conditions that A is finitely generated and F is not divisible by any polynomial of the above type are essentially necessary.
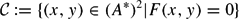\begin{equation*}
\mathcal{C}:=\{ (x,y)\in (A^*)^2 | F(x,y)=0 \}
\end{equation*}
- Type
- Research Article
- Information
- Mathematical Proceedings of the Cambridge Philosophical Society , Volume 158 , Issue 2 , March 2015 , pp. 331 - 353
- Copyright
- Copyright © Cambridge Philosophical Society 2015
References
REFERENCES
[1]Aschenbrenner, M.Ideal membership in polynomial rings over the integers. J. Amer. Math. Soc. 17 (2004), 407–442.CrossRefGoogle Scholar
[2]Bérczes, A., Evertse, J.-H. and Győry, K.Effective results for hyper- and superelliptic equations over number fields. Publ. Math. Debrecen 82 (2013), 727–756.Google Scholar
[3]Bérczes, A., Evertse, J.-H. and Győry, K.Effective results for Diophantine equations over finitely generated domains. Acta Arith. 163 (2014), 71–100.Google Scholar
[4]Bérczes, A., Evertse, J.-H., Győry, K. and Pontreau, C.Effective results for points on certain subvarieties of tori, Math. Proc. Camb. Phil. Soc. 147 (2009), 69–94.Google Scholar
[5]Bombieri, E. and Gubler, W., Heights in Diophantine Geometry (Cambridge University Press, Cambridge, 2006).Google Scholar
[6]Brindza, B.On the equation f(x) = ym over finitely generated domains. Acta Math. Hungar. 53 (1989), 377–383.Google Scholar
[7]Brindza, B.The Catalan equation over finitely generated integral domains. Publ. Math. Debrecen 42 (1993), 193–198.Google Scholar
[8]Brindza, B. and Pintér, Á.On equal values of binary forms over finitely generated fields. Publ. Math. Debrecen 46 (1995), 339–347.CrossRefGoogle Scholar
[9]Brindza, B., Pintér, A. and Végső, J.The Schinzel–Tijdeman equation over function fields. C.R. Math. Rep. Acad. Sci. Canada 16 (1994), 53–57.Google Scholar
[10]Brownawell, W. D. and Masser, D. W.Vanishing sums in function fields. Math. Proc. Camb. Phil. Soc. 100 (1986), 427–434.Google Scholar
[11]Bugeaud, Y. and Győry, K.Bounds for the solutions of unit equations. Acta Arith. 74 (1996), 67–80.Google Scholar
[12]Evertse, J.-H. and Győry, K.Effective results for unit equations over finitely generated integral domains. Math. Proc. Camb. Phil. Soc. 154 (2013), 351–380.Google Scholar
[13]Győry, K.Sur les polynômes à coefficients entiers et de dicriminant donné II. Publ. Math. Debrecen 21 (1974), 125–144.CrossRefGoogle Scholar
[14]Győry, K.On the number of solutions of linear equations in units of an algebraic number field. Comment. Math. Helv. 54 (1979), 583–600.Google Scholar
[15]Győry, K.Bounds for the solutions of norm form, discriminant form and index form equations in finitely generated integral domains. Acta Math. Hungar. 42 (1983), 45–80.Google Scholar
[16]Győry, K.Effective finiteness theorems for polynomials with given discriminant and integral elements with given discriminant over finitely generated domains. J. Reine Angew. Math. 346 (1984), 54–100.Google Scholar
[17]Győry, K. and Yu, K.Bounds for the solutions of S-unit equations and decomposable form equations. Acta Arith. 123 (2006), 9–41.CrossRefGoogle Scholar
[18]Hermann, G.Die Frage der endlich vielen Schritte in der Theorie der Polynomideale. Math. Ann. 95 (1926), 736–788.Google Scholar
[19]Hindry, M. and Silverman, J. H.Diophantine Geometry. Graduate Texts in Mathematics, vol. 201 (Springer-Verlag, New York, 2000).Google Scholar
[20]Lang, S. Integral points on curves. Inst. Hautes Études Sci. Publ. Math. (1960), 27–43.Google Scholar
[21]Louboutin, S.Explicit bounds for residues of Dedekind zeta functions, values of L-functions at s = 1, and relative class numbers. J. Number Theory 85 (2000), 263–282.Google Scholar
[22]Mahler, K.Zur Approximation algebraischer Zahlen. I. Math. Ann. 107 (1933), 691–730.Google Scholar
[23]Parry, C. J.The p-adic generalisation of the Thue–Siegel theorem. Acta Math. 83 (1950), 1–100.Google Scholar